Number System in Computer

The number system is something we use every day to complete our tasks and routine activities. For this, we have a system in place with unique symbols and specific values. This system becomes what we call a number system helping us calculate everything.

The basic features of a number system are – unique symbols, consistency, comparable value results and are easy to reproduce. The Decimal system remains the most basic system used by humans with a base power of 10 but the same things can’t go for machines as well.

Machines interpret numbers differently from humans thus require a different system altogether. Whenever we type something on a device, those letters convert into certain numbers which only the computer can understand. A positional number system is what a device needs where there are digits and different values making up an entire number. Each digit has its own unique position and a symbol. Each digit’s value can be determined by – the digit, position of the digit in the number, and the base of the number.

Table of Contents

Types of Number System

There are four number systems that a computer supports. They are –

Binary Number System
Decimal Number System
Octal Number System
Hexadecimal Number System

S. No.	Number System	Description	Example
1	Binary Number System	Base 2. Digits used: 0, 1	111010
2	Decimal Number System	Base 10. Digits used: 0 to 9	978
3	Octal Number System	Base 8. Digits used: 0 to 7	16527
4	Hexadecimal Number System	Base 16. Digits used: 0 to 9 Letters used: A- F	12FC

Binary Number System

Binary Number System consists of 2 digits i.e. 0 and 1. Therefore, it has a base value 2. All the digital computers use binary number system and converts the decimal number format to binary equivalent.

Every number that we see on a computer is an electrical signal which was previously represented by on and off creating a binary switch. The on and off-state uses 1 and 0 to represent the situation making the binary number system a base of 2.

Only these two symbols represent every number. 0 is for the lower rate while 1 is for the higher rate. The numbers are not as an individual unit here but are made of groups of 1s and 0s. Each binary digit is a bit and the place values are ascending powers of two from left to right. The least significant bit is on the rightmost side while the most significant bit is on the leftmost side. For example – 11010

When converting to decimal system –
= 11010
= 1×24 + 1×23 + 0×22 + 1×21 + 0×20
= 16 + 8 + 0 + 2 + 0
= 2610

Decimal to Binary Conversion

Divide the decimal number by 2
Keep the integer quotient for the coming iteration
Keep the remainder for the binary digit
Repeat the steps till you get 0 as your quotient

Example –

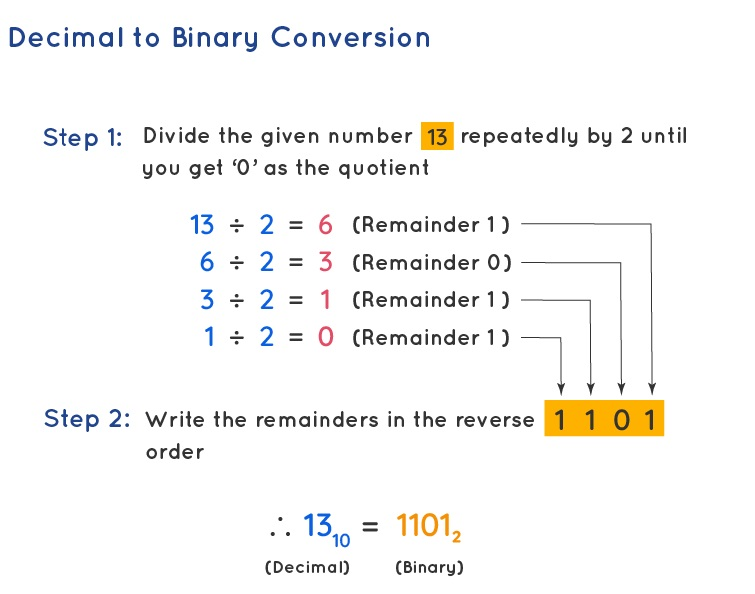
Division	Remainder (R)
13 / 2 = 6	1
6 / 2 = 3	0
3 / 2 = 1	1
1 / 2 = 0	1

Decimal Number System

The decimal number system is the most common number system we use in our daily lives. This number system has only 10 digits as it uses numbers from 0 and ends at 9. All digits have their own place value according to their position.

With each movement from right to left, the number value increases by 10. The position on the left of the decimal is for tens, hundreds, thousands, and so on units.

For example – The decimal number 4567 consists of 7 at the unit position, 6 in the tens position, 2 in the hundreds position, and 1 in the thousands position.

(4 x 1000)+ (5 x 100)+ (6 x 10)+ (7 x l)
4000 + 500 + 60 + 7
4567

Octal Number System

Octal Number System consists of 8 digits from 0 to 7.Therefore, it has a base value 8.

The last digit of an octal number has the x power of the base. This number system is not very common and used mostly when the number of bits is a multiple of 3. UNIX system and UTF8 numbers use it as a shorthand for file representation.

The reason behind the development of this system was to make binary more compact. It makes binary digits in a group of three instead of four. The base system of eight comes from the decimal number system only. For example – 125708
When converting to the decimal system –

125708
= ((1 x 84) + (2 x 83) + (5 x 82) + (7 x 81) + (0 x 80))10
= (4096 + 1024 + 320 + 56 + 0)10
= 549610

Binary to Octal Conversion

The first step is to group the binary digits in the set of 3.
Multiple each group by adding zeros to make it divisible by 3.
Write an octal symbol for each group underneath.
This will give you an octal number that arrived from a binary number.
Reversing the last two steps will give a binary number from an octal one.

Example – 1010111100
= (1010111100)2
= (001 010 111 100)2
= (1 2 7 4)8
= (1274)8

Hexadecimal Number System

Hexadecimal Number System consists of 16 digits from Oto 9 and the letters A to F. A- F represents numbers 10 to 15. It has a base value 16. It has a base value 16 because hex = 6 and Decimal = 10 and combining them makes the hexadecimal = 16.

In order to overcome this issue, the hexadecimal number system evolved putting binary numbers into a group of four bits. It is a compact approach to represent numbers on the computer as it requires only 4 bits. The hexadecimal number system has a base of 16 which means it uses 16 symbols in total.

This system has 10 numbers of the decimal system and A, B, C, D, E, and F as extra symbols. These letters are just to represent numbers that come after 10. Every place value in the number stands for 0 power of the base while the last digits have the z power of the base. For example – 27FB

When converting to decimal system –

27FB × 16 = 2 × 16 3 + 7×16 2 + 15×16 1 + 10×16

= 8192 + 1792 + 240 +10

= 1023410

Binary to Hexadecimal Conversion

The first step is to group the binary digits in the set of 4.
And then each quartet gets replaced by hexadecimal representation.
This will give you a hexadecimal number that arrived from a binary number.

Example – 1010101101001

= (1010101101001)2
= (1 0101 0110 1001)2
= (0001 0101 0110 1001)2
= (1 5 6 9)16
= (1569)16

Number System Relationship

HEXADECIMAL	DECIMAL	OCTAL	BINARY
0	0	0	0000
1	1	1	0001
2	2	2	0010
3	3	3	0011
4	4	4	0100
5	5	5	0101
6	6	6	0110
7	7	7	0111
8	8	10	1000
9	9	11	1001
A	10	12	1010
B	11	13	1011
C	12	14	1100
D	13	15	1101
E	14	16	1110
F	15	17	1111

Number Conversion

Computer – KnowledgeSthali